June 25, 2023
This is about an apparent paradox in probability called the Shooting Room, though it’s also known by other names. Surprisingly, there doesn’t seem to be a Wikipedia article about it (yet), so I’ll explain how it works.
Thrust into a room, you are assured that 90% of those who enter it will be shot. Panic! But you then learn that you will leave the room alive unless double-six is thrown, first time, with two dice. How is this compatible with the assurance that 90% will be shot? Successive batches of people thrust into the room are successively larger, exponentially, so that the forecast “90% will be shot” will be confirmed when double-six is eventually thrown. If knowing this, and knowing also that the dice falls would be utterly unpredictable even to a Laplacean demon who knew everything about the situation when the dice were thrown, then shouldn’t one’s panic vanish?
“Vanish” is clearly the wrong word, but that’s beside the point. This is the earliest reference I could find to the Shooting Room. It was part of an article about the Doomsday Argument, which I will not talk about here. My only concern is the apparent paradox. The exact numbers can vary, but the important part is the question of how likely you are to die, given that you’re chosen: Is the chance high or low?
The Shooting Room requires an infinite number of people sorted in some order. We don’t know our position in that order, and we can’t give it a uniform prior. So either we need some knowledge about our position, or the whole thing falls apart.
I’m not the first to think of this objection. Paul Bartha and Christopher Hitchcock have thought of it, among others I’m sure. Some people have tried to solve the paradox by taking a limit of truncated shooting rooms, where you can run out of people and have to stop early. The argument goes that you have a low chance of dying in each truncated room (assuming you’re chosen at all), so you have a low chance of dying in the original room (assuming you’re chosen). But this doesn’t actually solve the problem.
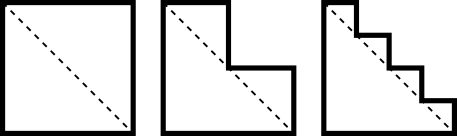
I’ve never seen anybody actually justify why you can take the limit of truncated rooms, and I strongly suspect it can’t be justified. To see why it needs justification, let’s use an analogy. Take a square, and fold in the top-right corner to the center. Then fold the two newly created corners onto the diagonal. Repeat this forever and you’ll get a triangle.

These stairstep shapes sure seem to be “approaching” the triangle in some sense. Does it follow that we can “take the limit” and conclude that the length of the hypotenuse is the sum of the lengths of the legs? No, of course not. Likewise with the Shooting Room. The truncated rooms seem to be “approaching” the original room, but it does not then follow that we can take the limit of probabilities from the truncated rooms.
In the truncated rooms, you have a high likelihood of being in the final group (again, assuming you play). If we were to “take the limit” again, we would have to conclude that you have a high likelihood of being in the final group of the original room. But that means dying. So the specious limit only appeared to solve the problem.
Suppose the people running the room roll the dice in advance. This doesn’t change anything; being unknown to us, the rolls are still random variables. Assuming \(N\) people are needed, suppose \(N - 1\) people are found and we’re added to this group. The group is placed in a uniformly random order and everything proceeds as usual.
This is a variant of the Shooting Room which involves no limit and no uniform prior over a countably infinite space. If we knew how many dice rolls there were, we would have a uniform belief about our position in the order. At first this seems to work, but there’s a problem. If we are told we’re in the first group (or second or third etc.) then we still expect to die with high probability. This seems to violate the spirit of the original room.
The Shooting Room seems paradoxical because it’s attempting to build an impossible probability space. No such thing can exist in theory or reality. When we ask what is our chance of dying, it is the same as asking what is the sum of the angles of a four-sided triangle. The answer is MU.